Durer's Melencolia I (1514) and The Magic Square
As we were studying Dürer's Saint Jerome in His Study last week, I discovered that it was one of a set of three large prints he made in 1513–14 known as his Meisterstiche or "master engravings." Another of the three is called Melencolia I. (The third one is Knight, Death, and the Devil). The prints seem to correspond to the three kinds of virtue in medieval scholasticism—moral, theological, and intellectual. Melencolia, being the depiction of intellectual scholasticism, has many interesting elements -tools of geometry and architecture, the polyhedron truncated rhombohedron (which, by the way, is now known as "Durer's solid"), an hourglass, a scale or balance, and compass.
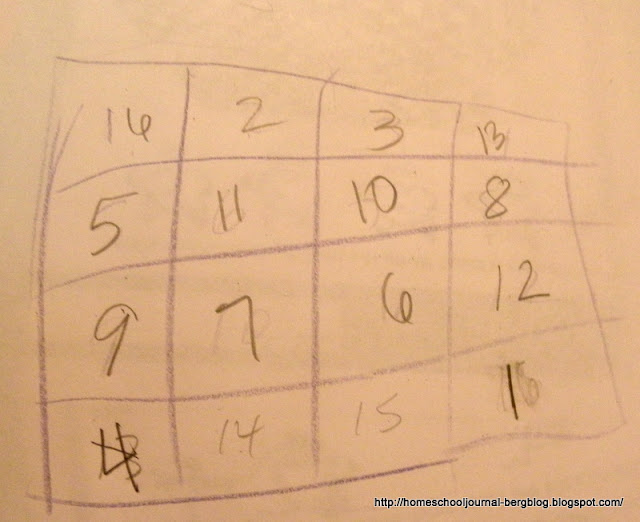
Perhaps the most interesting part of the engraving, however, is the "Magic Square" in the upper right hand corner of the engraving. A magic square is a grid of numbers in which you may find the same sum when adding the numbers within it in many different patterns and combinations. Durer's square is a 4 × 4 magic square with its rows horizontally, vertically, angles down, the four quadrants, (the four squares in each of the corners), corners and as well as the sum of the middle four numbers equal the same number, 34, which happens to belong to the Fibonacci sequence. You can even connect the numbers to form a parallelogram by connecting the numbers 3, 5, 14 and 12 to form 34. Another charming touch is that the numbers 15 and 14 appear in the middle of the bottom row, indicating the date of the engraving, 1514.
Then we made our own Magic Square.
First start by making a 4 × 4 grid and filling it in with the numbers 1-16 in order. Take the numbers in the diagonal from the top number 1 to the bottom number 16 and erase them and then fill them back in, in the opposite order.
Now do the same with the other diagonal -from 4-13.
Now start adding. See how many sums of 34 we get?
Then we compared it to Durer's square, to see how is it the same and how it is different?
Why don't you try it and see what you think?
Can you make more magic squares using the same method with different outcomes?