Place Value: Introduction: The Counting Game (Pre K-2)

Concept Development

"Zero spocks and one."

This counting game activity gives students a understanding of place value (base 10) by exploring groupings in bases other than ten and look for the patterns. Once they can see and understand the patterns of smaller groupings, it is easier to understand the same patterns in base ten.

I begin by asking for a silly word or fun game. This makes the game more appealing. Quentin suggested spock, but have your student come up with his own silly word. It can be a totally made up word like zurkle or bosco or bloop. I then explained the rules to the game. The first rule is that the blue column is where we are going to put spocks. The green column is where we add one counter at a time. As soon as we have enough for a Spock, we exchange the green counters for one blue spock counter. You determine in advance what base you want to explore of this day and explain to him that once you get to a certain amount, it becomes one of your silly words and goes in the blue column. In this case, I chose for us to explore base 4, so every time we had added one more to three in the green column, we exchange the four greens for 1 spock.. For each round, the student should read the board, saying the number of spocks first and then the number of ones. You can ring a bell to signal the start of a new round or you can just say "new round,' whichever would appeal to your student more.

The picture above is the result of round one, and Quentin read it as "zero spocks and one."

"Zero spocks and two."

This is the result of round two. Continue on in this way until you get to the base you want to explore.

"Zero spocks and three."

"Spock!"

"One spock, and zero."

 Make sure that your student exchanges the four counters for one spock.

Keep playing the game, counting one at a time up to the point that you would need to exchange the counters in the blue column for one in the next (red) column. Now start taking one away for each round. Make sure your student repeats the name for each step.

"Three spocks and two."

Play this game as long as you have his interest. The younger your student, the more days you might have to play it, but play it until your student can easily add and subtract by one and regroup without being instructed.

 Once you reach that point, you are ready to start the game all over again with a new  amount, and a new fun word. You can also change materials because this encourages flexible thinking.

Repeat the game with various groupings and names until they can anticipate the adding and subtracting.

After some time, perhaps weeks or even months, and your student has played several grouping games and you feel they really understand the pattern, proceed to connecting symbols to the concept.

Connecting Symbolization to the Concept

Using Base 5, we set our counters, with the line down the middle representing the same division as the blue and green divisions above. On this particular day, they were called "Googas.'

 We counted directly on the board with the ones on one side and the googas on the other.
We then wrote numerically on the side.

We also use number-flips, which can be as simple as homemade numeral cards made from index cards cut in half. As they count up and down the base they are practicing, they flip over to make the numeral match the amounts on their counting boards.

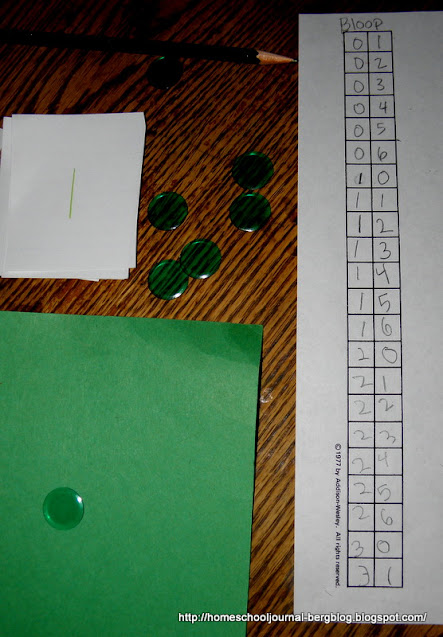
Using Symbols to Record the Concept

 Once you feel they can  confidently count up and down a variety of bases, you can begin the recording level of this game. Have them continue to add one, of whatever manipulative you wish to use, at a time to their boards, but instead of flipping to the appropriate numeral, ask them to read their boards and you can add this to a strip of 2-column block paper. Repeat this activity with several other base grouping and he should be able to do the recording page independently before long. When they finish a recording page, look together for patterns. 

To introduce variety in making written records, introduce a square array chart. If you are doing sixes, use a 6 x6 graph grid, if you are doing base four, use a 4 x 4 graph grid and so on.  Have them copy the sequence and then color the patterns they see, such as where do all the same numerals fall in the different place value columns? For example, where do all the 1's fall in the ones column or the bloops (or whatever word you are using) column?